
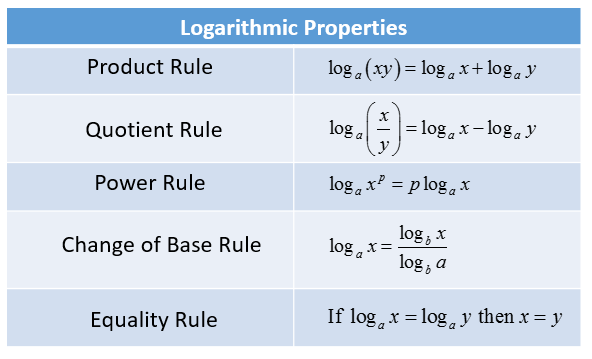
This can be any base b we want where b > 0, b ≠ 1. The Change-of-Base Formula introduces a new base b. y = log b M log b a Substitute y = log a M. log b a y = log b M Use the Power Property. y = log a M Rewrite the expression in exponential form. log a M = log b M log b a Suppose we want to evaluate log a M. To evaluate a logarithm with any other base, we can use the Change-of-Base Formula. Use the Properties of Logarithms to condense the logarithm 2 log x + 2 log ( x + 1 ) 2 log x + 2 log ( x + 1 ). 1 4 ( 3 log 2 x − log 2 3 − 2 log 2 y − log 2 z ) log 2 x 3 3 y 2 z 4 = 1 4 ( 3 log 2 x − log 2 3 − 2 log 2 y − log 2 z )
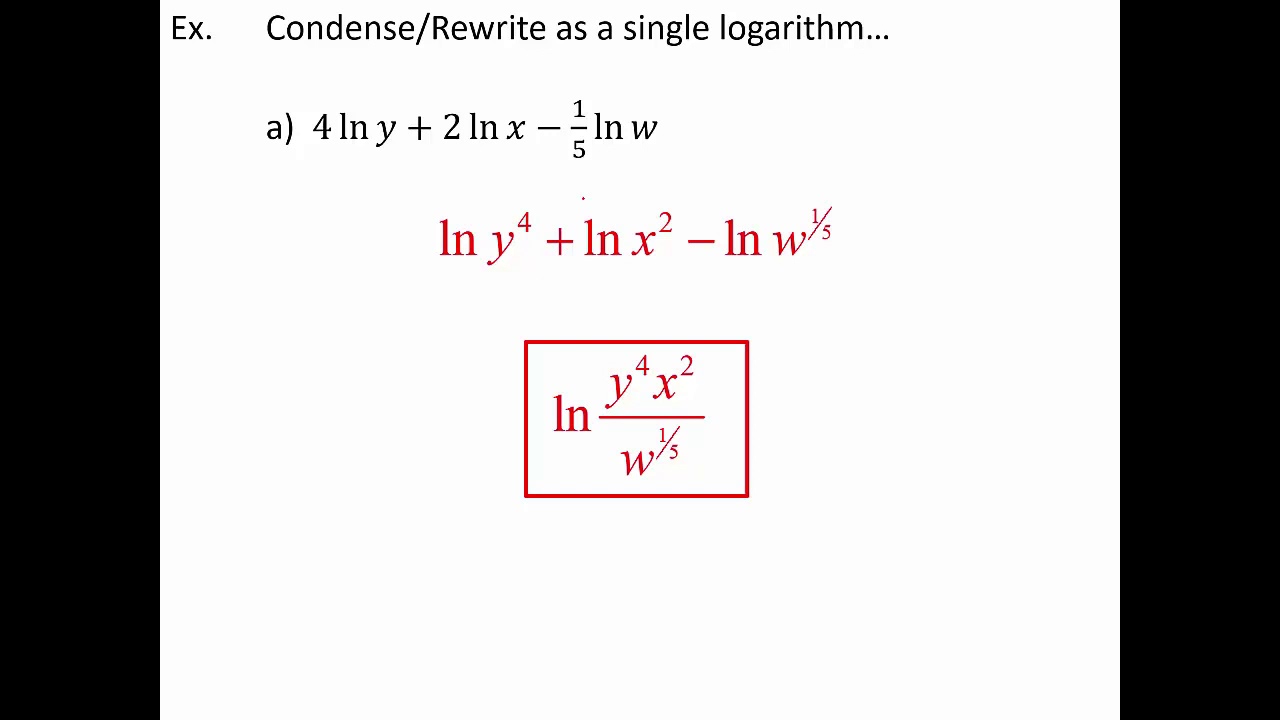
1 4 ( 3 log 2 x − ( log 2 3 + 2 log 2 y + log 2 z ) ) Simplify by distributing. 1 4 ( log 2 ( x 3 ) − ( log 2 3 + log 2 y 2 + log 2 z ) ) Use the Power Property, log a M p = p log a M, inside the parentheses.
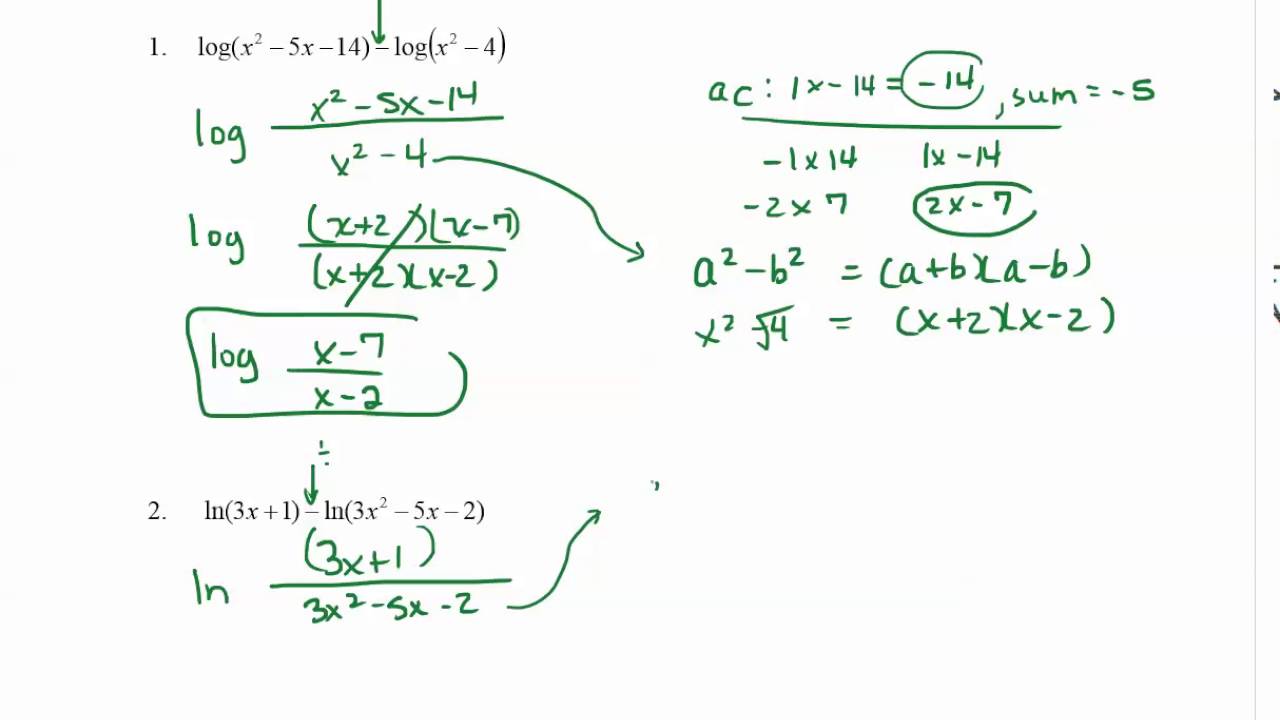


 0 kommentar(er)
0 kommentar(er)
